
|

Problem #1 The organizers of the Wonderland Olympics are trying to decide how many homes to build to house the Wonderland Athletes during the competition. The only stipulation is that each home must contain the same number of athletes. After the homes are built, the organizers realize that they cannot house the same number of athletes in each home. When they try to put six athletes into each home, one athlete is left over. All seems lost, when the organizers realize that one home needs to be used for the organizers themselves. With one home removed, the other athletes can now all fit into the remaining homes, each home now containing the same number of athletes. Assuming there remain at least two homes to house athletes, how many athletes are coming to the Wonderland Olympics? Problem #2  One of the events, the Obstacle Course, contains a 30 tile long section
with a moving conveyor belt. With the belt turned off, Stinky can run
the entire distance in just 12 seconds. With the belt turned on (and Stinky running against the direction of its movement), it
takes Stinky 30 seconds to run the distance. How long will it take
Stinky on the return trip, if the belt is still running in the same
direction? One of the events, the Obstacle Course, contains a 30 tile long section
with a moving conveyor belt. With the belt turned off, Stinky can run
the entire distance in just 12 seconds. With the belt turned on (and Stinky running against the direction of its movement), it
takes Stinky 30 seconds to run the distance. How long will it take
Stinky on the return trip, if the belt is still running in the same
direction?
Problem #3 In total, there were twelve events the Wonderland Olympics. Athletes came from three different “teams”: Wondertown, Forest’s End, and Wonderfalls. 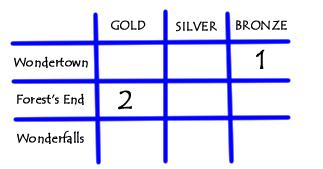 At the end of the competition, the organizers tabulated the number of
Gold, Silver, and Bronze medals that each team had won for all twelve
events. For example, Wondertown completed the Wonderland Olympics with
exactly one Bronze medal. Forest’s End came out with having won exactly
two Gold medals. At the end of the competition, the organizers tabulated the number of
Gold, Silver, and Bronze medals that each team had won for all twelve
events. For example, Wondertown completed the Wonderland Olympics with
exactly one Bronze medal. Forest’s End came out with having won exactly
two Gold medals.When all was said and done, the organizers noticed something odd. Each of the three teams won exactly the same number of medals, and no two numbers in the grid were the same. Can you figure out who won how many Gold, Silver, and Bronze medals in total?

|

